The Science Of
Musical Colors
“An innovative way to make music fun and easy to comprehend for children and people of all ages." - Gardner Cole
"If you are a beginner or an expert guitarist it doesn't matter, the Musical Colors stickers improve your knowledge and familiarity with the fretboard." - Antonio Davide Pino
"Musical Colors stickers open new possibilities in musical ideas when composing or improvising on the instrument. They are very helpful in growing as a musician!" - Ray Flores
“Easy to understand with a fun filled process. I would have been lost without it and paid for lessons.” - Anton Nathrass
“These stickers inspired and supported my creative and unique approach to song writing.” - The Wiley One
“I believe in this product, the guitar stickers helped me visually learn the fretboard with ease.” - Brian Howland
“A perfect aesthetic compliment, as well as opening up some doors theory wise and visually.” - Russell Anthony
“I just love improvising on my guitar and actually seeing all the patterns at my fingertips." - Michael Wiley
Dream it
“An innovative way to make music fun and easy to comprehend for children and people of all ages." - Gardner Cole "If you are a beginner or an expert guitarist it doesn't matter, the Musical Colors stickers improve your knowledge and familiarity with the fretboard." - Antonio Davide Pino "Musical Colors stickers open new possibilities in musical ideas when composing or improvising on the instrument. They are very helpful in growing as a musician!" - Ray Flores “Easy to understand with a fun filled process. I would have been lost without it and paid for lessons.” - Anton Nathrass “These stickers inspired and supported my creative and unique approach to song writing.” - The Wiley One “I believe in this product, the guitar stickers helped me visually learn the fretboard with ease.” - Brian Howland “A perfect aesthetic compliment, as well as opening up some doors theory wise and visually.” - Russell Anthony “I just love improvising on my guitar and actually seeing all the patterns at my fingertips." - Michael Wiley Dream it
The Whole Systems Synthesis
Of Music, Color and Geometry
A Scientific Deep Dive
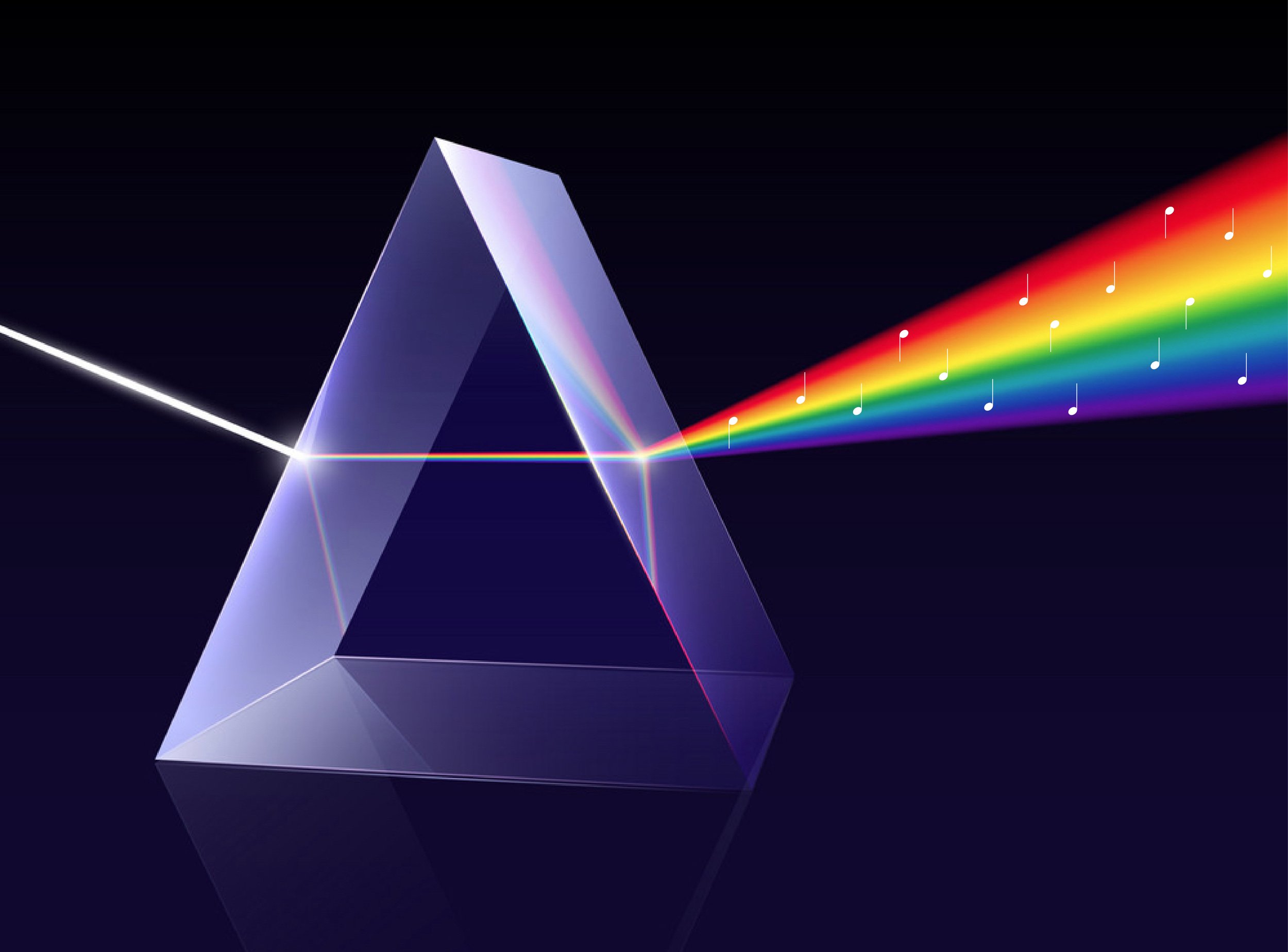
Sound And Color are Vibrations Of The Unified Field Found In Nature
We believe that bringing music and color together is one of the most natural things to do, just as a handful of great minds have sought to do so in recent and ancient times. We postulate that relatively soon there will be a universally accepted and standardized unification of these two very different but complimentary and proportionally similar artistic languages of music and color, yielding an all encompassing and expanding Unified Musical Colors Theory. In effect, a synthesis between the two disciplines, tied together by the mathematical equation:
VF=(((432^2)•1609.344)/(AF•(2)^40)•10^10)/10
-
We assert that frequencies (in hertz) of audible tone frequencies (AF) between 360Hz. and 720Hz. respectively, can be converted to wavelengths (in nano-meters) of visual color frequencies (VF), by transposing upward using the Law of Octaves (i.e. the doubling of audio frequencies repetitively), and divided into what we believe to nodally be the theoretical one-way Speed of Light in miles/second (432 squared, or 186,624 miles per second) converted to meters (i.e. multiplied by the number of meters 1609.344 in a mile). It is important to understand that the second is not just an ever changing arbitrary measurement based on atomic structure, it was originally based on the length of time it takes for a pendulum to make a complete swing through the cycle of an arc of 30º degrees, which is rooted in the origin of ancient measurements (including gravity) having been discovered and not invented by man. In this way, the 7 natural notes in music (A, B, C, D, E, F, & G) are mathematically and scientifically assigned color designations, all determined from their inherently similar proportions and by using the mathematical formula that is represented above.
The Natural Or True “Middle Octave” Found In Western Music
Actually begins One tritone up from middle C(c4) at the note f#4
-
Numerical figures above are rounded to the nearest whole number and are the result of taking the average of several tuning systems based on A4 = 432 cycles per second, comparatively including Pythagorean, Factor 9 and Equal Temperament tuning. Only the Natural or True "Middle Octave" in music is mathematically related to, and overlaps the Color Spectrum (i.e. f#4 - f5), which is flanked by the infrared (IR) and ultraviolet (UV) spectrums. The use of the color Violet for the f# above is shown for Musical Colors reference purposes only and does not imply that any Violet color exists at the bottom of the Color Spectrum, rather it visually shows how the musical note f at the top of the spectrum is linked together using the color violet, with the f# at the bottom of the spectrum, as this represents the cyclical repetition found in music. Naturals use solid colors and sharps and flats use the two colors of the adjacent natural note colors, side by side, in order to visually distinguish the sharps and flats from the naturals, rather than using the actual shade in between the two, which is impractical.
C Is The Center Of Music Just As Green lies at
The Center Of The Natural Light Color Spectrum
THE RAINBOW
The Synesthetic Brain Can See
Music And Hear Color? what!
Scientific inquiry has uncovered a compelling link between color and music, demonstrating shared mathematical proportions rooted in the natural order. This intersection is particularly evident in the harmonious correspondence between musical notes and the colors of the visible spectrum, where frequencies align with specific wavelengths. The phenomenon of synesthesia adds an extraordinary dimension, as some individuals seamlessly perceive both color and music in conjoined ways, offering insights into the intricate wiring of the human brain, which hints at the intertwined pathways responsible for processing these sensory experiences. Overall, the scientific exploration of color and music reveals an obvious and profound interconnectedness, from mathematical harmonies to the extraordinary perceptual symphonies experienced by a unique subset of individuals.
Studies show that color enhances memory retention throughout many disciplines and especially in music.
Some professional musicians have personal relationships between music and color which help them do what they do best.
Color coded music systems help to make a positive difference in music education for many children and adults alike.
-
From the dawn of humanity, the mesmerizing dance between music and color has created an intricate tapestry of artistic expression. This profound connection occasionally blossoms into the captivating realm of synesthesia—a neurological phenomenon that transcends the conventional boundaries of sensory perception. In synesthesia, the stimulation of one sensory pathway, such as the auditory experience of music, triggers an involuntary and simultaneous response in another pathway, often resulting in the vivid experience of seeing colors. Picture a symphony transforming into a burst of vibrant hues or a melancholic melody evoking a cascade of cool blues and purples. This extraordinary fusion of senses provides a unique lens through which individuals perceive and interpret the world around them.
Synesthesia, though not universally experienced, has been a subject of fascination for scientists, artists, and philosophers alike. The study of this phenomenon unveils the complex interplay of the brain's neural networks and the intriguing ways in which different sensory modalities can cross paths. Artists like Wassily Kandinsky, a renowned painter, claimed to "hear" colors, translating the auditory experience of music into visual masterpieces. For Kandinsky, a crescendo might manifest as a riot of warm, energetic reds and yellows, while a gentle melody could evoke serene, cool blues and greens on his canvas. These artists provide tangible examples of how synesthesia enriches the creative process, offering a unique and deeply personal dimension to their work.
As we delve into the profound impact of color, a vibrant mosaic of knowledge emerges. Countless volumes, diverse theories, and extensive research endeavors explore the intricate relationship between color and its effects on cognition, emotion, and aesthetics. Some of these explorations extend their reach into the realm of music, emphasizing the interconnectedness of these two expressive languages. The findings coalesce into a compelling consensus: color serves as a powerful cognitive tool, enhancing memory, simplifying complexity, and providing a visual structure amid the apparent chaos of our environment.
The language of color, with its nuanced palette, possesses the remarkable ability to induce both relaxation and active engagement, fostering deeply meaningful and enjoyable experiences. It acts as a visual symphony, guiding our emotional responses and shaping the narratives of our encounters with art and the world. In this sense, color becomes a silent yet potent storyteller, influencing the way we perceive, interpret, and remember the multifaceted tapestry of human experiences.
In essence, color is a facilitator of a rich, nuanced relationship with the world, often overlooked in the hustle and bustle of our daily lives. Beyond its aesthetic appeal, color becomes a key player in the profound connection between music and human experience. The ways in which color is creatively harnessed to expedite musical learning and enrich individual musical experiences form an integral part of contemporary music education. These practices serve as a testament to the enduring and innovative fusion of color and music, representing a collective journey toward a deeper understanding and appreciation of the sensory wonders that shape our perception of the world.
How do color, shape & Sound
Mathematically Fit together?
-
The table below should initially be read from the bottom up, starting at what is known as the fundamental primal shape in nature, the Triangle. F#3 or 180Hz. is the infrared frequency at where the 8 Euclidian Shapes, the 5 Platonic Solids, and their respective partnering and increasingly complex geometries begin to emanate, adhering to a natural hierarchy of mathematical proportion found in nature. The sums of the interior angles of these shapes individually, when expressed as cycles per second in Hertz, give rise to a mathematical adherence to the proportions of the natural Harmonic Overtone Series (i.e. demonstrated by the partial numbers 1-20 on the right side of the table) and the Golden Mean, Sacred Cut or Ratio, also known as Phi (1.618…). This sacred never ending number is the most irrational number known to man, because it represents the eternal search for perfection. The Natural Light Spectrum is nicely nested just above the infrared spectrum, between the first octave F#4 360Hz in the series. progressing upward, and terminating before reaching the ultraviolet spectrum, the third octave F#5 720Hz. Remember to start at the bottom of this table to see how it all mathematically builds up.
THE NATURAL COLOR-SHAPE-
SOUND CORRELATION TABLE
The Higher Complex Solids
______________________________________________________________________
42480˚ Truncated Icosidodecahedron
E^11 42480Hz. 4th Major 7th
20880˚ Truncated Dodecahedron,
Truncated icosahedron, Rhombicosi-
dodecahedron & Snub Dodecahedron
E10 20880Hz. 3rd Major 7th
16560˚ Truncated Cuboctahedron
C10 16560Hz. 2nd Diminished
5th / Augmented 4th / Tritone
10080˚ Icosidodecahedron
D#9 10080Hz. 2nd Major 6th
7920˚ Truncated Octahedron,
Truncated cube, , Snub Cube
& Rhombicuboctahedron
B8 7920Hz. 1st Perfect 4th
The Upper Platonic Solids
______________________________________________________________________
6480˚ Dodecahedron G#8
6480Hz. 3rd Major 2nd
3600˚ Icosahedron Truncated
Tetrahedron / Cuboctahedron A#7
3600Hz. 3rd M 3rd (20)
(3456) A7 3456Hz. Minor 3rd (19)
(3240) G#7 3240Hz. 2nd Major 2nd (18)
(3096) G7 3060Hz. minor 2nd (17)
(2880) F#7 2880Hz. 4th Octave (16)
(2736) F7 2736Hz. Major 7th (15)
(2592) E7 2592Hz. 2nd minor 7th (14)
(2448) D#7 2340Hz. Major 6th (13)
The Mid Platonic Solid &
4th Part of F# Major Harmony
F# 1440Hz. A# 1800Hz. C# 2160Hz.
______________________________________________________________________
2160˚ Hexahedron (Cube) C#7
2160Hz. 3rd Perfect 5th (12)
(2070) C7 2070Hz. 1st Diminished
5th/Augmented 4th/Tritone (11)



















